Uniswap V3
Uniswap V2 采用 CFMM 恒定函数做市商算法,池子的流动性是分布在无穷区域上的,池子允许在任何价格的交易发生,从 0 到正无穷。但一个资产的历史价格通常是在某个区间内的,不管这个区间是大还是小,在远离当前价格区间的、永远不会达到的某个点上提供流动性是毫无意义的。
Uniswap V3 引入了 集中流动性(concentrated liquidity) 的概念:LP 可以选择他们希望在哪个价格区间提供流动性。这个机制通过将更多的流动性提供在一个相对狭窄的价格区间,大大提高了资产利用效率。
Uniswap V3 的 tick 之间的工作机制跟 V2 完全一样,tick 之间的流动性是可以完全耗尽的。
下面了解几个公式:
- L = \sqrt{xy} 池子中的流动性是两种 token 资产数量的组合。两种代币数量乘积为 k,因此我们可以用 \sqrt{xy} 来衡量池子流动性。L 实际上是 x 和 y 的几何平均数。
- \sqrt{P}= \sqrt{\frac{x}{y}} 这里 \frac{x}y 是 token0 相对于 token1 的价格。
- 输出数量 ∆y 在交易中满足 L=\frac{∆y}{∆\sqrt{P}},这里 ∆y 是交易中 token y 变化的数量,∆\sqrt{P} 是交易中 \sqrt{P} 变化的数量。这个公式成立的根本是交易行为不能导致 L 发生变化,\sqrt{xy} = \sqrt{x_0 y_0} = \sqrt{x_1 y_1} 交易前后 L 不变,详见 Uniswap V3 Book。
- 因为双方的价格互为倒数,所以 输入数量 ∆x = ∆\frac{1}{\sqrt{P}}L
Ticks
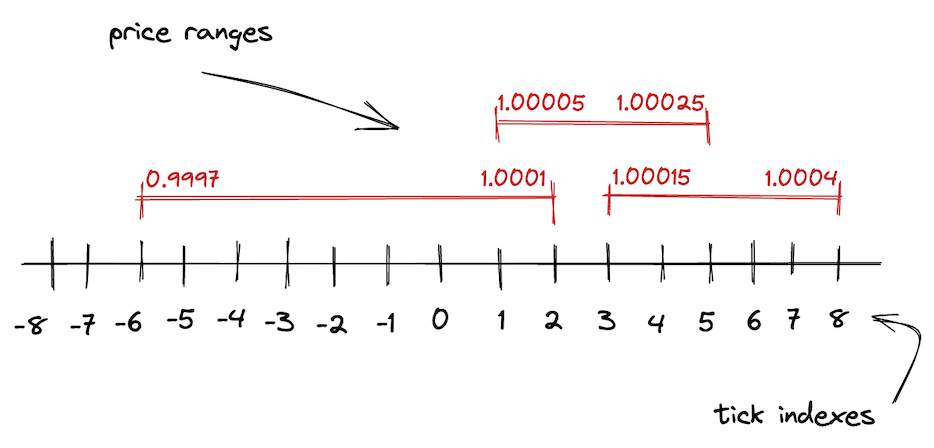
Ticks 是 V3 中的一大核心,V2 中的无穷价格区间在 V3 中被分成了更小的价格区间,每个区间都由上下界端点进行限制。为了进行这些边界的协调,V3 引入了 ticks。价格不能落在两个相邻的 tick 之间,只能落到 tick 上。
每个 tick 有一个 index 和对应的价格:p(i) = 1.0001^i,p(i) 即为 tick i 的价格。但因为 Uniswap V3 储存的是 \sqrt{P} 而不是 P,所以这个公式实际是 \sqrt{p(i)}=\sqrt{1.0001^i} = 1.0001^{\frac{i}{2}},所以他的值大概是 \sqrt{p(0)}=1,\sqrt{p(1)}≈1.00005,\sqrt{p(-1)}≈0.99995。
在 V3 中 \sqrt{P} 使用 Q64.96 储存,受限于数据长度,能够保存的价格范围是 [-2^{2*64},2^{2*64}],所以对应的 ticks 的取值范围为 [log_{1.0001}2^{-128},log_{1.0001}2^{128}] = [−887272, 887272]。
计算流动性
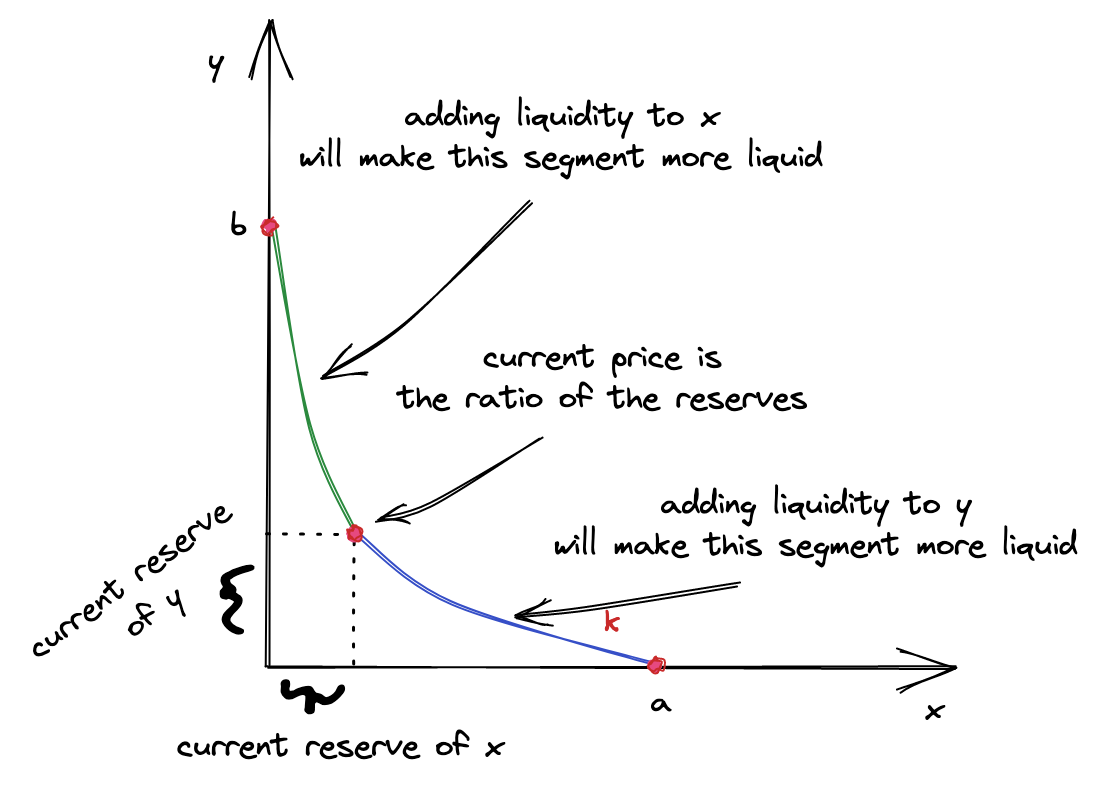
Liquidity 计算
根据之前的公式 结合上图计算流动性 L 的值,
将公式 ∆x=∆\frac{1}{\sqrt{P}}L 展开一下 ∆x=(\frac{1}{\sqrt{P_c}} - \frac{1}{\sqrt{P_b}})L 则 L=∆x\frac{\sqrt{P_b}\sqrt{P_c}}{\sqrt{P_b}-\sqrt{P_c}}
将公式 ∆y=(\sqrt{P_c} - \sqrt{P_a})L 则 L=\frac{∆y}{\sqrt{P_c}-\sqrt{P_a}}
重新计算 token 数量
因为我们使用 ∆x 和 ∆y 计算出了两个不同的 L,我们会用小的那个。所以最初用来计算的 ∆x 和 ∆y 需要代入上一步的公式中使用小的 L 重新计算 ∆x 和 ∆y 这是最终用户会添加进去的流动性。
Swap 计算
比如我们要实现一个 Uniswap V3 like 的 DEX 的聚合器,Swap 的 input / output amount 的计算是绕不过去的,最简单的思路就是通过 RPC 调用 Quote 合约查询 output amount,但是这里有一个弊端就是通过 Quote 合约查询的结果是跨多个 tick 执行完成后的最终结果。这种情况下我们没法知道 pool 的每个 tick 的流动性,比如想要将 10000 A 换成 B,pool 中在价格
- tick1: 100 A = 1 B 处有 10 B 流动性
- tick2: 150 A = 1 B 处有 10 B 流动性
- tick3: 200 A = 1 B 处有 40 B 流动性
所以 tick1 用 1000A 换了 10B 还 9000A,然后 tick2 1500A 换了 10B 还 7500A,tick3 7500A 换了 37.5B 兑换完成,quote 合约返回了一个 57.5B 的结果。设想一下如果还有一个路径是 A->C->B,它的计算后价格是 110 A = 1 B 这个价格下的流动性有 40 B,你如何在不知道每个 tick 下流动性的情况下严谨的拆分订单?
所以要想知道每个 tick 下的流动性,有两种方法,
- 部署一个修改版 Quote 合约,把每个 tick 上的流动性也返回回来。
- 拿到一些基础的数据,比如
slot0中的当前价格、流动性,即当前价格的position对应的当前及上下一位wordPos的 bitmap 数据,即对应已初始化 tick 的TickInfo,通过其他语言在链下实现相同的逻辑,也可以准确的计算出 output amount。
奶爸为你趟了一遍坑,结论是可行的,通过用 go 重新实现了 tick、tick math、liquidity math、full math、bitmap 合约中的一些方法,奶爸成功在链下仅用几个链上数据实现了 exactInput 的 output amount 的计算。
inputToken := common.HexToAddress("0xA0b86991c6218b36c1d19D4a2e9Eb0cE3606eB48")
outputToken := common.HexToAddress("0x853d955aCEf822Db058eb8505911ED77F175b99e")
amountIn := big.NewInt(19000000000000)
token0, token1 := sortToken(&inputToken, &outputToken)
println("token0", token0.String())
println("token1", token1.String())
isZerForOne := token0 == &inputToken
println("isZerForOne", isZerForOne)
client, err := ethclient.Dial("https://cloudflare-eth.com")
if err != nil {
panic(err)
}
pool, err := contracts.NewUniswapV3Pool(common.HexToAddress("0xc63b0708e2f7e69cb8a1df0e1389a98c35a76d52"), client)
if err != nil {
panic(err)
}
fee, err := pool.Fee(nil)
if err != nil {
panic(err)
}
token0FromPool, err := pool.Token0(nil)
if err != nil {
panic(err)
}
token1FromPool, err := pool.Token1(nil)
if err != nil {
panic(err)
}
if token0FromPool != *token0 || token1FromPool != *token1 {
panic("token0 or token1 is not match")
}
callOpts := &bind.CallOpts{
BlockNumber: big.NewInt(19760163),
}
liquidity, err := pool.Liquidity(callOpts)
if err != nil {
panic(err)
}
tickSpacing, err := pool.TickSpacing(callOpts)
if err != nil {
panic(err)
}
slot0, err := pool.Slot0(callOpts)
if err != nil {
panic(err)
}
quoterV2, err := contracts.NewUniswapV3QuoterV2(common.HexToAddress("0x61fFE014bA17989E743c5F6cB21bF9697530B21e"), client)
if err != nil {
panic(err)
}
sqrtPriceMin, err := v3utils.GetSqrtRatioAtTick(v3utils.MaxTick - 1)
if err != nil {
panic(err)
}
ret, err := quoterV2.QuoteExactInputSingle(callOpts, contracts.IQuoterV2QuoteExactInputSingleParams{
TokenIn: inputToken,
TokenOut: outputToken,
AmountIn: amountIn,
Fee: fee,
SqrtPriceLimitX96: sqrtPriceMin,
})
if err != nil {
panic(err)
}
fmt.Printf("on-chain ret: %+v\n", ret)
var state SwapState
state.AmountSpecifiedRemaining = new(big.Int).Set(amountIn)
state.SqrtPriceX96 = slot0.SqrtPriceX96
state.Liquidity = liquidity
state.Tick = slot0.Tick
state.AmountCalculated = big.NewInt(0)
for !lib.IsZero(state.AmountSpecifiedRemaining) && lib.Ne(state.SqrtPriceX96, sqrtPriceMin) == 1 {
fmt.Printf("\n\n\n======== amountRemaining: %d =========\n", state.AmountSpecifiedRemaining.Int64())
var step StepComputations
step.SqrtRatioStartX96 = state.SqrtPriceX96
tickmapKey := lib.GetTickMapKeyForTick(int(slot0.Tick.Int64()), int(tickSpacing.Int64()), isZerForOne)
tickMap := make(map[int]*big.Int)
for i := int(tickmapKey) - 1; i <= int(tickmapKey)+1; i++ {
v, err := pool.TickBitmap(callOpts, int16(i))
if err != nil {
panic(err)
}
tickMap[i] = v
}
fmt.Printf("tickMap: %+v\n", tickMap)
step.Initilized, step.TickNext = lib.NextInitializedTickWithinOneWord(tickMap, int(state.Tick.Int64()), int(tickSpacing.Int64()), isZerForOne)
fmt.Printf("nextTickInitialized: %t, nextTick: %d\n", step.Initilized, step.TickNext)
if step.TickNext < v3utils.MinTick {
step.TickNext = v3utils.MinTick
} else if step.TickNext > v3utils.MaxTick {
step.TickNext = v3utils.MaxTick
}
step.SqrtPriceNextX96, err = lib.GetSqrtRatioAtTick(step.TickNext)
if err != nil {
panic(err)
}
var sqrtRatioTargetX96 *big.Int
var flag bool
if isZerForOne {
flag = lib.Gt(step.SqrtPriceNextX96, state.SqrtPriceX96) == 1
} else {
flag = lib.Gt(step.SqrtPriceNextX96, sqrtPriceMin) == 1
}
if flag {
sqrtRatioTargetX96 = sqrtPriceMin
} else {
sqrtRatioTargetX96 = step.SqrtPriceNextX96
}
state.SqrtPriceX96, step.AmountIn, step.AmountOut, step.FeeAmount, err = v3utils.ComputeSwapStep(state.SqrtPriceX96, sqrtRatioTargetX96, state.Liquidity, state.AmountSpecifiedRemaining, constants.FeeAmount(fee.Uint64()))
if err != nil {
panic(err)
}
fmt.Printf("sqrtRatioNext: %s, retAmtIn: %s, amtOut: %s, feeAmt: %s\n", state.SqrtPriceX96.String(), step.AmountIn.String(), step.AmountOut.String(), step.FeeAmount.String())
fmt.Printf("state: %+v\n", state)
// if exactInput {
state.AmountSpecifiedRemaining.Sub(state.AmountSpecifiedRemaining, new(big.Int).Add(step.AmountIn, step.FeeAmount))
state.AmountCalculated.Sub(state.AmountCalculated, step.AmountOut)
// } else {
// TODO: exactOutput
// }
if lib.Ne(state.SqrtPriceX96, step.SqrtPriceNextX96) == 0 {
if step.Initilized {
tickInfo, err := pool.Ticks(callOpts, big.NewInt(int64(step.TickNext)))
if err != nil {
panic(err)
}
liquidityNet := tickInfo.LiquidityNet
if isZerForOne {
liquidityNet.Neg(liquidityNet)
}
state.Liquidity = lib.AddDelta(state.Liquidity, liquidityNet)
}
if isZerForOne {
state.Tick.SetInt64(int64(step.TickNext) - 1)
} else {
state.Tick.SetInt64(int64(step.TickNext))
}
} else if lib.Ne(state.SqrtPriceX96, step.SqrtPriceNextX96) == 1 {
tick, err := lib.GetTickAtSqrtRatio(state.SqrtPriceX96)
if err != nil {
panic(err)
}
state.Tick.SetInt64(int64(tick))
}
}
var amount0, amount1 *big.Int
// if isZerForOne == exactInput {
amount0 = new(big.Int).Sub(amountIn, state.AmountSpecifiedRemaining)
amount1 = new(big.Int).Set(state.AmountCalculated)
// } else {
// TODO: exactOutput
// }
fmt.Printf("\n\n\nisZeroForOne: %t, amountIn: %d, state: %+v\n", isZerForOne, amountIn, state)
fmt.Printf("amount0: %s, amount1: %s\n", amount0.String(), amount1.String())
if amount1.CmpAbs(ret.AmountOut) != 0 {
panic("amount1 is not match")
}
核心就在 Pool 合约中的 swap 方法,一步一步实现即可,下面是执行后的效果。
token0 0x853d955aCEf822Db058eb8505911ED77F175b99e
token1 0xA0b86991c6218b36c1d19D4a2e9Eb0cE3606eB48
isZerForOne false
on-chain ret: {AmountOut:+18996651967770371746104874 SqrtPriceX96After:+79262835938196828630617 InitializedTicksCrossed:3 GasEstimate:+144147}
======== amountRemaining: 19000000000000 =========
2024/05/06 22:47:47 tick = -276340, tickSpacing = 10, zeroForOne = false
2024/05/06 22:47:47 compressed = -27634
tickMap: map[-109:+25711008708143844408671393477458601640355247900524685364822016 -108:+633825300114114700748472448192 -107:+0]
nextTickInitialized: true, nextTick: -276330
sqrtRatioNext: 79204503519858955838074, retAmtIn: 7327321285028, amtOut: 7335092912385649064434176, feeAmt: 3665493390
state: {AmountSpecifiedRemaining:+19000000000000 AmountCalculated:+0 SqrtPriceX96:+79204503519858955838074 Liquidity:+15842738790231437704582 Tick:-276340}
======== amountRemaining: 11669013221582 =========
2024/05/06 22:47:51 tick = -276330, tickSpacing = 10, zeroForOne = false
2024/05/06 22:47:51 compressed = -27633
tickMap: map[-109:+25711008708143844408671393477458601640355247900524685364822016 -108:+633825300114114700748472448192 -107:+0]
nextTickInitialized: true, nextTick: -276320
sqrtRatioNext: 79244113692861321940131, retAmtIn: 7919825073883, amtOut: 7920596115673192183308463, feeAmt: 3961893484
state: {AmountSpecifiedRemaining:+11669013221582 AmountCalculated:-7335092912385649064434176 SqrtPriceX96:+79244113692861321940131 Liquidity:+15841213013653411008977 Tick:-276330}
======== amountRemaining: 3745226254215 =========
2024/05/06 22:47:53 tick = -276320, tickSpacing = 10, zeroForOne = false
2024/05/06 22:47:53 compressed = -27632
tickMap: map[-109:+25711008708143844408671393477458601640355247900524685364822016 -108:+633825300114114700748472448192 -107:+0]
nextTickInitialized: true, nextTick: -276310
sqrtRatioNext: 79262835938196828630617, retAmtIn: 3743353641087, amtOut: 3740962939711530498362235, feeAmt: 1872613128
state: {AmountSpecifiedRemaining:+3745226254215 AmountCalculated:-15255689028058841247742639 SqrtPriceX96:+79262835938196828630617 Liquidity:+15840996916216165493682 Tick:-276320}
isZeroForOne: false, amountIn: 19000000000000, state: {AmountSpecifiedRemaining:+0 AmountCalculated:-18996651967770371746104874 SqrtPriceX96:+79262835938196828630617 Liquidity:+15840996916216165493682 Tick:-276316}
amount0: 19000000000000, amount1: -18996651967770371746104874
结果是当我们知道了 pool 中每个 tick 的流动性,我们可以合理的将订单拆分。
- 4400 A -> C -> 40 B
- 4600 A -> 37.5 B
最终我们通过合理拆分订单可以多得到 20 B,当然实际的情况比这个要复杂,本文旨在探索 off-chain 计算的可行性。